Page 90 - Mathematics Class - XI

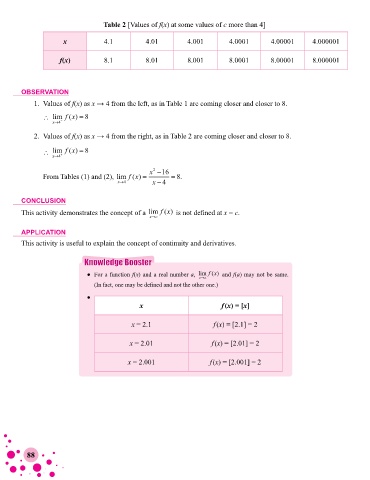
Table 2 [Values of f(x) at some values of c more than 4]

x 4.1 4.01 4.001 4.0001 4.00001 4.000001

f(x) 8.1 8.01 8.001 8.0001 8.00001 8.000001

OBSERVATION
1. Values of f(x) as x → 4 from the left, as in Table 1 are coming closer and closer to 8.

\ lim( )fx = 8
x→4 −
2. Values of f(x) as x → 4 from the right, as in Table 2 are coming closer and closer to 8.

\ lim( )fx = 8
x→4 +

2
From Tables (1) and (2), lim( )fx = x −16 = . 8
x→4 x − 4

CONCLUSION

This activity demonstrates the concept of a lim( )fx is not defined at x = c.
x→ c

APPLICATION
This activity is useful to explain the concept of continuity and derivatives.

Knowledge Booster

● For a function f(x) and a real number a, lim( )fx and f(a) may not be same.
x→ a
(In fact, one may be defined and not the other one.)
●
x f (x) = [x]

x = 2.1 f (x) = [2.1] = 2

x = 2.01 f (x) = [2.01] = 2

x = 2.001 f (x) = [2.001] = 2

88