Page 86 - Mathematics Class - XI

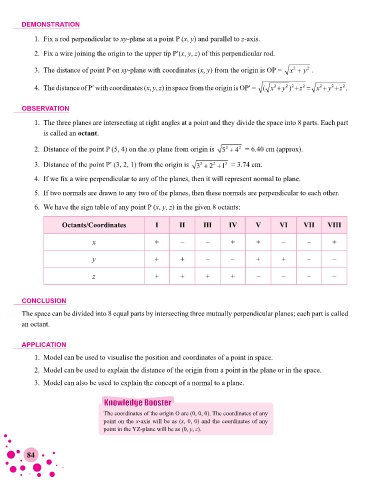
DEMONSTRATION

1. Fix a rod perpendicular to xy-plane at a point P (x, y) and parallel to z-axis.

2. Fix a wire joining the origin to the upper tip P′(x, y, z) of this perpendicular rod.

2
2
3. The distance of point P on xy-plane with coordinates (x, y) from the origin is OP = x + y .
4. The distance of P′ with coordinates (x, y, z) in space from the origin is OP′ = ( x + y 2 2 z = x + y + z 2 .
) +
2
2
2
2
OBSERVATION
1. The three planes are intersecting at right angles at a point and they divide the space into 8 parts. Each part
is called an octant.

2. Distance of the point P (5, 4) on the xy plane from origin is 5 + 4 = 6.40 cm (approx).
2
2
3. Distance of the point P′ (3, 2, 1) from the origin is 3 + 2 2 + 2 1 = 3.74 cm.
2
4. If we fix a wire perpendicular to any of the planes, then it will represent normal to plane.

5. If two normals are drawn to any two of the planes, then these normals are perpendicular to each other.

6. We have the sign table of any point P (x, y, z) in the given 8 octants:

Octants/Coordinates I II III IV V VI VII VIII

x + – – + + – – +

y + + – – + + – –

z + + + + – – – –

CONCLUSION

The space can be divided into 8 equal parts by intersecting three mutually perpendicular planes; each part is called
an octant.

APPLICATION

1. Model can be used to visualise the position and coordinates of a point in space.
2. Model can be used to explain the distance of the origin from a point in the plane or in the space.

3. Model can also be used to explain the concept of a normal to a plane.

Knowledge Booster
The coordinates of the origin O are (0, 0, 0). The coordinates of any
point on the x-axis will be as (x, 0, 0) and the coordinates of any
point in the YZ-plane will be as (0, y, z).

84