Page 94 - Mathematics Class - X

PROJECT 7

AIM
To prove Pythagoras theorem (by method other than given in textbook).

MATERIALS REQUIRED

A piece of cardboard
Two sheets of white paper

A pair of scissors

PROCEDURE
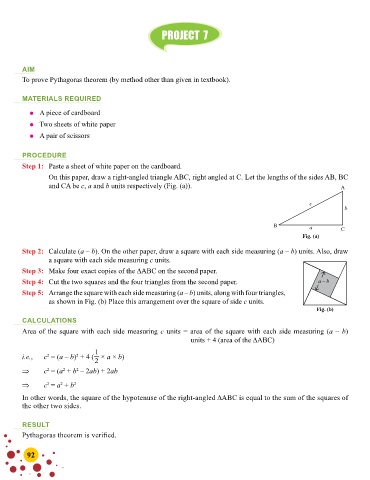
Step 1: Paste a sheet of white paper on the cardboard.

On this paper, draw a right-angled triangle ABC, right angled at C. Let the lengths of the sides AB, BC
and CA be c, a and b units respectively (Fig. (a)). A

c
b

B a C
Fig. (a)

Step 2: Calculate (a – b). On the other paper, draw a square with each side measuring (a – b) units. Also, draw
a square with each side measuring c units.
Step 3: Make four exact copies of the ∆ABC on the second paper.
Step 4: Cut the two squares and the four triangles from the second paper. a – b
Step 5: Arrange the square with each side measuring (a – b) units, along with four triangles,
as shown in Fig. (b) Place this arrangement over the square of side c units.
Fig. (b)
CALCULATIONS
Area of the square with each side measuring c units = area of the square with each side measuring (a – b)
units + 4 (area of the ∆ABC)

1
i.e., c = (a – b) + 4 ( × a × b)
2
2
2
⇒ c = (a + b – 2ab) + 2ab
2
2
2
⇒ c = a + b
2
2
2
In other words, the square of the hypotenuse of the right-angled ∆ABC is equal to the sum of the squares of
the other two sides.
RESULT
Pythagoras theorem is verified.

92