Page 64 - Mathematics Class - X

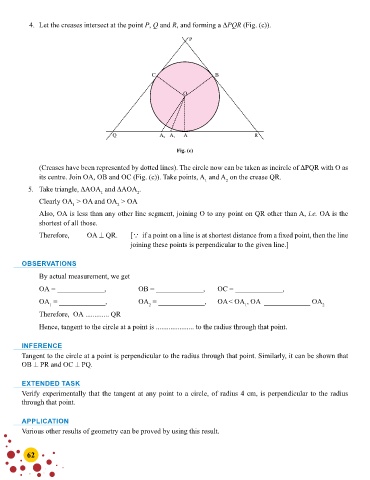
4. Let the creases intersect at the point P, Q and R, and forming a ∆PQR (Fig. (c)).

Fig. (c)

(Creases have been represented by dotted lines). The circle now can be taken as incircle of ∆PQR with O as
its centre. Join OA, OB and OC (Fig. (c)). Take points, A and A on the crease QR.
1 2
5. Take triangle, ∆AOA and ∆AOA .
1 2
Clearly OA > OA and OA > OA
1 2
Also, OA is less than any other line segment, joining O to any point on QR other than A, i.e. OA is the
shortest of all those.

Therefore, OA ⊥ QR. [Q if a point on a line is at shortest distance from a fixed point, then the line
joining these points is perpendicular to the given line.]

OBSERVATIONS

By actual measurement, we get
OA = _____________, OB = _____________, OC = _____________,

OA = _____________, OA = _____________, OA< OA , OA _____________ OA
1 2 1 2
Therefore, OA ............. QR
Hence, tangent to the circle at a point is ..................... to the radius through that point.

INFERENCE
Tangent to the circle at a point is perpendicular to the radius through that point. Similarly, it can be shown that
OB ⊥ PR and OC ⊥ PQ.

EXTENDED TASK
Verify experimentally that the tangent at any point to a circle, of radius 4 cm, is perpendicular to the radius
through that point.

APPLICATION
Various other results of geometry can be proved by using this result.

62