Page 90 - Physics - XI

Result

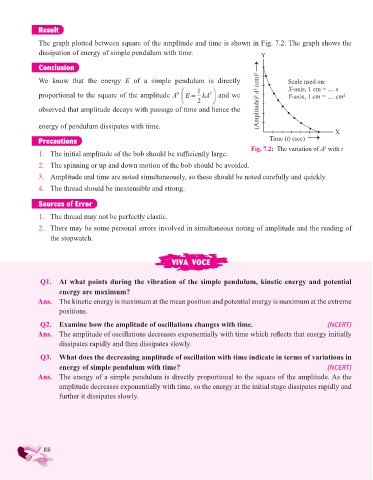
The graph plotted between square of the amplitude and time is shown in Fig. 7.2. The graph shows the
dissipation of energy of simple pendulum with time. Y

Conclusion

We know that the energy E of a simple pendulum is directly Scale used on:

proportional to the square of the amplitude A 2 E 1 kA 2 and we X-axis, 1 cm = .... s 2
Y-axis, 1 cm = .... cm
2 (Amplitude) 2 A 2 (cm) 2
observed that amplitude decays with passage of time and hence the

energy of pendulum dissipates with time.
X
Precautions Time (t) (sec)
Fig. 7.2: The variation of A with t
2
1. The initial amplitude of the bob should be suffi ciently large.
2. The spinning or up and down motion of the bob should be avoided.
3. Amplitude and time are noted simultaneously, so these should be noted carefully and quickly.

4. The thread should be inextensible and strong.

Sources of Error
1. The thread may not be perfectly elastic.

2. There may be some personal errors involved in simultaneous noting of amplitude and the reading of
the stopwatch.

VIVA VOCE

Q1. At what points during the vibration of the simple pendulum, kinetic energy and potential
energy are maximum?
Ans. The kinetic energy is maximum at the mean position and potential energy is maximum at the extreme
positions.

Q2. Examine how the amplitude of oscillations changes with time. (NCERT)
Ans. The amplitude of oscillations decreases exponentially with time which refl ects that energy initially
dissipates rapidly and then dissipates slowly.

Q3. What does the decreasing amplitude of oscillation with time indicate in terms of variations in
energy of simple pendulum with time? (NCERT)
Ans. The energy of a simple pendulum is directly proportional to the square of the amplitude. As the
amplitude decreases exponentially with time, so the energy at the initial stage dissipates rapidly and
further it dissipates slowly.

88