Page 88 - Physics - XI

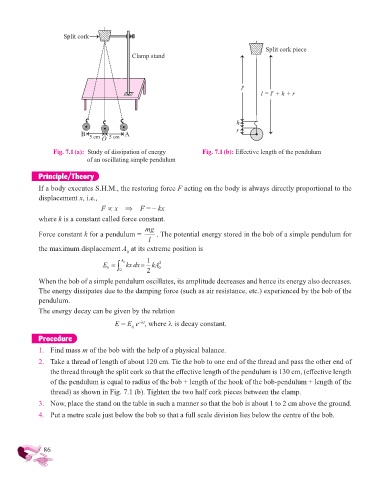
Split cork
Split cork piece
Clamp stand

l′
l = l′ + h + r

h
r
B 5 cm O 5 cm A

Fig. 7.1 (a): Study of dissipation of energy Fig. 7.1 (b): Eff ective length of the pendulum
of an oscillating simple pendulum

Principle/Theory

If a body executes S.H.M., the restoring force F acting on the body is always directly proportional to the
displacement x, i.e.,
F ∝ x ⇒ F = – kx
where k is a constant called force constant.
mg
Force constant k for a pendulum = . The potential energy stored in the bob of a simple pendulum for
l
the maximum displacement A at its extreme position is
0

0
E A 0 kxdx 1 kA 2
0 2 0
When the bob of a simple pendulum oscillates, its amplitude decreases and hence its energy also decreases.
The energy dissipates due to the damping force (such as air resistance, etc.) experienced by the bob of the
pendulum.
The energy decay can be given by the relation

E = E e , where λ is decay constant.
–λt
0
Procedure

1. Find mass m of the bob with the help of a physical balance.
2. Take a thread of length of about 120 cm. Tie the bob to one end of the thread and pass the other end of
the thread through the split cork so that the eff ective length of the pendulum is 130 cm, (eff ective length
of the pendulum is equal to radius of the bob + length of the hook of the bob-pendulum + length of the

thread) as shown in Fig. 7.1 (b). Tighten the two half cork pieces between the clamp.
3. Now, place the stand on the table in such a manner so that the bob is about 1 to 2 cm above the ground.
4. Put a metre scale just below the bob so that a full scale division lies below the centre of the bob.

86