Page 29 - Physics - XI

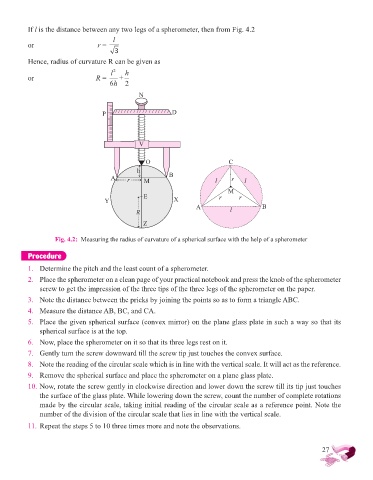
If l is the distance between any two legs of a spherometer, then from Fig. 4.2
l
or r =
3
Hence, radius of curvature R can be given as
l 2 h
or R = +
6 h 2
N

P D

V

O C
h
A r M B l r l
M
E r r
Y X
A B
R l
Z

Fig. 4.2: Measuring the radius of curvature of a spherical surface with the help of a spherometer

Procedure

1. Determine the pitch and the least count of a spherometer.
2. Place the spherometer on a clean page of your practical notebook and press the knob of the spherometer
screw to get the impression of the three tips of the three legs of the spherometer on the paper.
3. Note the distance between the pricks by joining the points so as to form a triangle ABC.
4. Measure the distance AB, BC, and CA.

5. Place the given spherical surface (convex mirror) on the plane glass plate in such a way so that its
spherical surface is at the top.
6. Now, place the spherometer on it so that its three legs rest on it.
7. Gently turn the screw downward till the screw tip just touches the convex surface.

8. Note the reading of the circular scale which is in line with the vertical scale. It will act as the reference.
9. Remove the spherical surface and place the spherometer on a plane glass plate.
10. Now, rotate the screw gently in clockwise direction and lower down the screw till its tip just touches
the surface of the glass plate. While lowering down the screw, count the number of complete rotations
made by the circular scale, taking initial reading of the circular scale as a reference point. Note the
number of the division of the circular scale that lies in line with the vertical scale.

11. Repeat the steps 5 to 10 three times more and note the observations.

27