Page 27 - Physics - XI

EXPERIMENT - 4

Aim

To determine radius of curvature of a given spherical surface by a spherometer.

Apparatus and Materials Required

A spherometer, a plane glass plate, a spherical surface (convex mirror), and a pencil

Description of the Measuring Device
Spherometer is a device which measures the radius of curvature of a spherical surface.

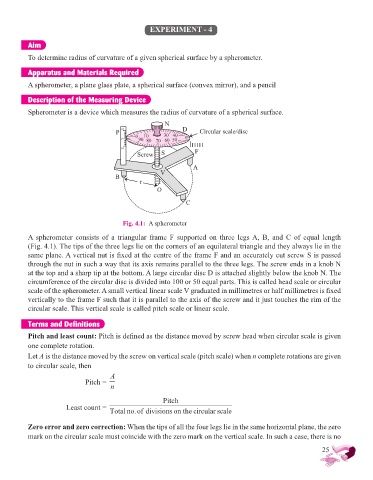
N
P 10 10 30 40 D Circular scale/disc
0 0 90 80 70 60 50
10 F
Screw S
A
V
B
r
O

C

Fig. 4.1: A spherometer

A spherometer consists of a triangular frame F supported on three legs A, B, and C of equal length
(Fig. 4.1). The tips of the three legs lie on the corners of an equilateral triangle and they always lie in the
same plane. A vertical nut is fi xed at the centre of the frame F and an accurately cut screw S is passed
through the nut in such a way that its axis remains parallel to the three legs. The screw ends in a knob N
at the top and a sharp tip at the bottom. A large circular disc D is attached slightly below the knob N. The
circumference of the circular disc is divided into 100 or 50 equal parts. This is called head scale or circular
scale of the spherometer. A small vertical linear scale V graduated in millimetres or half millimetres is fi xed
vertically to the frame F such that it is parallel to the axis of the screw and it just touches the rim of the
circular scale. This vertical scale is called pitch scale or linear scale.

Terms and Definitions

Pitch and least count: Pitch is defi ned as the distance moved by screw head when circular scale is given
one complete rotation.
Let A is the distance moved by the screw on vertical scale (pitch scale) when n complete rotations are given
to circular scale, then
A
Pitch =
n

Pitch
Least count =
Total no. of divisions on the circular scale

Zero error and zero correction: When the tips of all the four legs lie in the same horizontal plane, the zero
mark on the circular scale must coincide with the zero mark on the vertical scale. In such a case, there is no

25