Page 120 - Physics - XI

8. Note all the observations in the observation table. Find (θ – θ ) for each observation and compute
0
log (θ – θ ) for each observation.
0

Observations
Temperature recorded by the thermometer A = T = ______ °C
1
Temperature recorded by the thermometer B = T = ______ °C
2
Correction to be applied for thermometer B = (T – T ) = ______ °C
1 2
Initial corrected temperature of enclosure = θ = ______ °C
1
Final corrected temperature of enclosure = θ = ______ °C
2
θ + θ
Mean corrected temperature of enclosure θ = 1 2 2 = ______ °C
0

Table for calculation of cooling with time

S. No. Time t (min) Temperature of hot water θθ (°C) (θ (θ – θθ ) (°C) log (θθ – θθ )
0 0
1.
2.
3.
4.
5.

6.
7.
8.
9.
10.

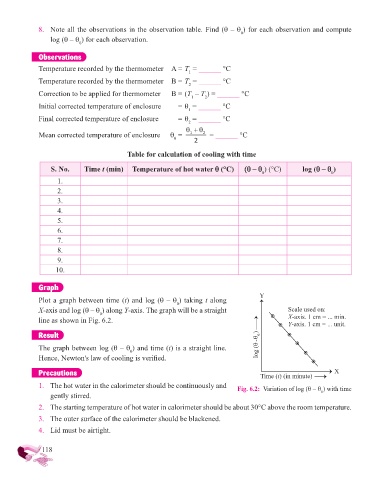
Graph
Y
Plot a graph between time (t) and log (θ – θ ) taking t along
0
X-axis and log (θ – θ ) along Y-axis. The graph will be a straight Scale used on:
0
line as shown in Fig. 6.2. X-axis. 1 cm = ... min.
Y-axis. 1 cm = ... unit.
Result
The graph between log (θ – θ ) and time (t) is a straight line. log (θ-θ 0 )
0
Hence, Newton's law of cooling is verifi ed.

Precautions Time (t) (in minute) X
1. The hot water in the calorimeter should be continuously and Fig. 6.2: Variation of log (θ – θ ) with time
gently stirred. 0

2. The starting temperature of hot water in calorimeter should be about 30°C above the room temperature.
3. The outer surface of the calorimeter should be blackened.

4. Lid must be airtight.

118