Page 69 - Maths Skills - 8

Cubes and Cube Roots 67

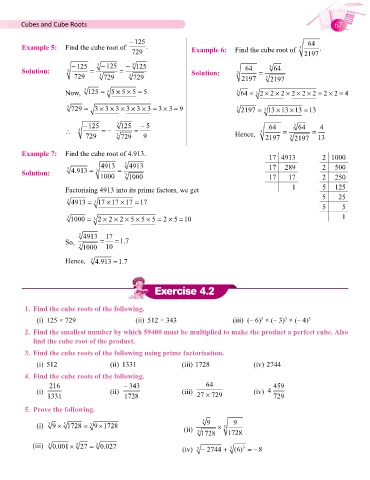
−125 64
Example 5: Find the cube root of . Example 6: Find the cube root of 3 .
729 2197

3
Solution: 3 −125 = 3 −125 = − 125 Solution: 3 64 = 3 64
729 3 729 3 729 2197 3 2197

Now, 3 125 = 3 555× ×= 5 3 64 = 3 2 ××××× 2 =×= 4
2
2
2
2
2
2

3 729 = 333333××××× =×= 3 2197 = 13 13 13 13× × =
339
3
−125 3 125 − 5 64 3 64 4
∴ 3 =− = Hence, 3 = =
729 3 729 9 2197 3 2197 13
Example 7: Find the cube root of 4.913.
17 4913 2 1000
Solution: 3 4 913. = 3 4913 = 3 4913 17 289 2 500
1000 3 1000 17 17 2 250
Factorising 4913 into its prime factors, we get 1 5 125
5 25
3 4913 = 3 17 17 17× × = 17
5 5
25 10=
3 1000 = 3 2 ×× 2 555××× =× 1
2

3 4913 17
So, = = 17.
3
1000 10
Hence, 4 913 17. = .
3

Exercise 4.2

1. Find the cube roots of the following.
(i) 125 × 729 (ii) 512 × 343 (iii) (– 6) × (– 3) × (– 4) 3
3
3
2. Find the smallest number by which 59400 must be multiplied to make the product a perfect cube. Also
find the cube root of the product.

3. Find the cube roots of the following using prime factorisation.
(i) 512 (ii) 1331 (iii) 1728 (iv) 2744
4. Find the cube roots of the following.

216 − 343 64 459
(i) (ii) (iii) 27 × (iv) 4
1331 1728 729 729
5. Prove the following.

3 9 9
3
3
(i) 9 1728 3 9 1728 (ii) 3 × 3
1728 1728
(iii) 3 0 001. 3 27 3 0 027. (iv) 3 2744 () 3 8
3
6