Page 65 - Maths Skills - 8

Cubes and Cube Roots 63

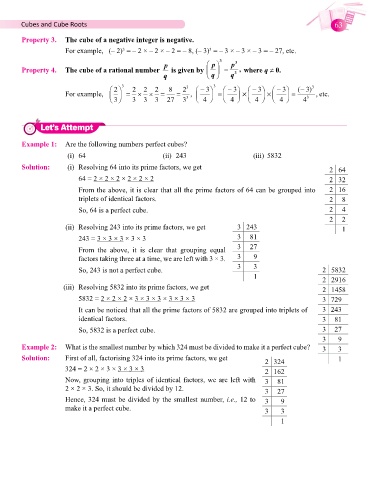
Property 3. The cube of a negative integer is negative.
For example, (– 2) = – 2 × – 2 × – 2 = – 8, (– 3) = – 3 × – 3 × – 3 = – 27, etc.
3
3
3 3
p p p
Property 4. The cube of a rational number is given by 3 , where q ≠ 0.
q q q

For example, 2 3 2 2 8 2 3 ,  −  3  3 =   −  3  ×  −  3  ×  −  3  = ( − 3) 3 ,etc.




3 3 3 3 27 3 3  4   4   4   4  4 3
Let’s Attempt

Example 1: Are the following numbers perfect cubes?
(i) 64 (ii) 243 (iii) 5832
Solution: (i) Resolving 64 into its prime factors, we get 2 64

64 = 2 × 2 × 2 × 2 × 2 × 2 2 32
From the above, it is clear that all the prime factors of 64 can be grouped into 2 16
triplets of identical factors. 2 8
So, 64 is a perfect cube. 2 4
2 2
(ii) Resolving 243 into its prime factors, we get 3 243 1
243 = 3 × 3 × 3 × 3 × 3 3 81
From the above, it is clear that grouping equal 3 27
factors taking three at a time, we are left with 3 × 3. 3 9

So, 243 is not a perfect cube. 3 3 2 5832
1 2 2916
(iii) Resolving 5832 into its prime factors, we get 2 1458
5832 = 2 × 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3 3 729

It can be noticed that all the prime factors of 5832 are grouped into triplets of 3 243
identical factors. 3 81
So, 5832 is a perfect cube. 3 27
3 9
Example 2: What is the smallest number by which 324 must be divided to make it a perfect cube? 3 3

Solution: First of all, factorising 324 into its prime factors, we get 2 324 1
324 = 2 × 2 × 3 × 3 × 3 × 3 2 162
Now, grouping into triples of identical factors, we are left with 3 81
2 × 2 × 3. So, it should be divided by 12. 3 27
Hence, 324 must be divided by the smallest number, i.e., 12 to 3 9
make it a perfect cube. 3 3

1