Page 178 - Maths Skills - 8

176 Maths

Dimensions of the Cuboid

The length, breadth (or width) and height (thickness or depth) are called the dimensions of the cuboid. We know
that the opposite sides of a rectangle are equal, so we have

AB = DC = EF = HG = l, the length of the cuboid, ED = FC = HA = GB = b, the breadth of the cuboid, and
AD = HE = BC = GF = h, the height of the cuboid.

SURFACE AREA OF A CUBOID AND CUBE
A cuboid has six rectangular faces, i.e., three opposite and equal faces, so its surface area will be equal to the sum
of the areas of six rectangular faces.

Surface Area of a Cuboid
The sum of the areas of the six faces of a cuboid is called the total surface area or simply the surface area of
a cuboid.
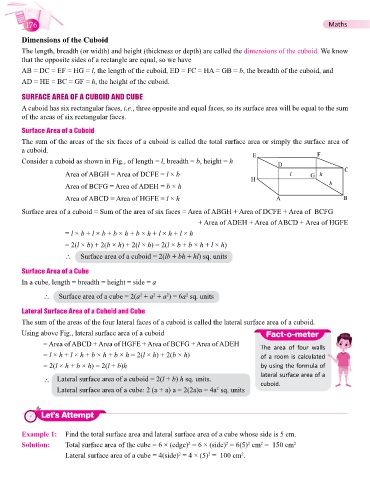
Consider a cuboid as shown in Fig., of length = l, breadth = b, height = h

Area of ABGH = Area of DCFE = l × b l h
Area of BCFG = Area of ADEH = b × h b

Area of ABCD = Area of HGFE = l × h

Surface area of a cuboid = Sum of the area of six faces = Area of ABGH + Area of DCFE + Area of BCFG
+ Area of ADEH + Area of ABCD + Area of HGFE
= l × b + l × b + b × h + b × h + l × h + l × h
= 2(l × b) + 2(b × h) + 2(l × h) = 2(l × b + b × h + l × h)

\ Surface area of a cuboid = 2(lb + bh + hl) sq. units

Surface Area of a Cube
In a cube, length = breadth = height = side = a

\ Surface area of a cube = 2(a + a + a ) = 6a sq. units
2
2
2
2
Lateral Surface Area of a Cuboid and Cube
The sum of the areas of the four lateral faces of a cuboid is called the lateral surface area of a cuboid.
Using above Fig., lateral surface area of a cuboid Fact-o-meter
= Area of ABCD + Area of HGFE + Area of BCFG + Area of ADEH The area of four walls
= l × h + l × h + b × h + b × h = 2(l × h) + 2(b × h) of a room is calculated
= 2(l × h + b × h) = 2(l + b)h by using the formula of
lateral surface area of a
\ Lateral surface area of a cuboid = 2(l + b) h sq. units. cuboid.

2
Lateral surface area of a cube: 2 (a + a) a = 2(2a)a = 4a sq. units

Let’s Attempt

Example 1: Find the total surface area and lateral surface area of a cube whose side is 5 cm.

Solution: Total surface area of the cube = 6 × (edge) = 6 × (side) = 6(5) cm = 150 cm 2
2
2
2
2
Lateral surface area of a cube = 4(side) = 4 × (5) = 100 cm .
2
2
2