Page 174 - Maths Skills - 8

172 Maths

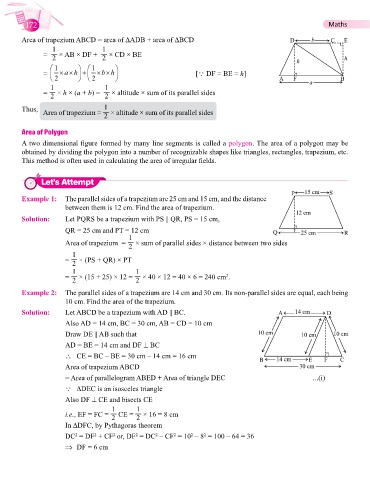
Area of trapezium ABCD = area of ∆ADB + area of ∆BCD b
1 1
= × AB × DF + × CD × BE h h
2
2
1 1
bh

= ah [ DF = BE = h]
2 2 a
1 1
= 2 × h × (a + b) = × altitude × sum of its parallel sides
2
1
Thus, Area of trapezium = × altitude × sum of its parallel sides
2

Area of Polygon
A two dimensional figure formed by many line segments is called a polygon. The area of a polygon may be
obtained by dividing the polygon into a number of recognizable shapes like triangles, rectangles, trapezium, etc.
This method is often used in calculating the area of irregular fields.

Let’s Attempt

Example 1: The parallel sides of a trapezium are 25 cm and 15 cm, and the distance
between them is 12 cm. Find the area of trapezium.
Solution: Let PQRS be a trapezium with PS || QR, PS = 15 cm,
QR = 25 cm and PT = 12 cm
1
Area of trapezium = × sum of parallel sides × distance between two sides
2
1
= 2 × (PS + QR) × PT

1 1
= 2 × (15 + 25) × 12 = × 40 × 12 = 40 × 6 = 240 cm .
2
2
Example 2: The parallel sides of a trapezium are 14 cm and 30 cm. Its non-parallel sides are equal, each being
10 cm. Find the area of the trapezium.
Solution: Let ABCD be a trapezium with AD || BC.

Also AD = 14 cm, BC = 30 cm, AB = CD = 10 cm
Draw DE || AB such that
AD = BE = 14 cm and DF ⊥ BC
\ CE = BC – BE = 30 cm – 14 cm = 16 cm

Area of trapezium ABCD
= Area of parallelogram ABED + Area of triangle DEC ...(i)
 ∆DEC is an isosceles triangle
Also DF ⊥ CE and bisects CE
1 1
i.e., EF = FC = CE = × 16 = 8 cm
2
2
In ∆DFC, by Pythagoras theorem
DC = DF + CF or, DF = DC – CF = 10 – 8 = 100 – 64 = 36
2
2
2
2
2
2
2
2
⇒ DF = 6 cm