Page 136 - Maths Skills - 8

134 Maths

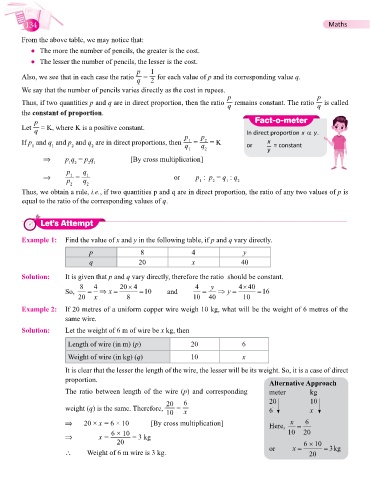
From the above table, we may notice that:
● The more the number of pencils, the greater is the cost.

● The lesser the number of pencils, the lesser is the cost.
p 1
Also, we see that in each case the ratio = for each value of p and its corresponding value q.
q
2
We say that the number of pencils varies directly as the cost in rupees.
p p
Thus, if two quantities p and q are in direct proportion, then the ratio remains constant. The ratio is called
q
q
the constant of proportion.
p Fact-o-meter
Let = K, where K is a positive constant. In direct proportion x a y.
q
If p and q and p and q are in direct proportions, then p 1 = p 2 = K x
1 1 2 2 q q or = constant
1 2 y
⇒ p q = p q [By cross multiplication]
2 1
1 2
p q
⇒ p 1 = q 1 or p : p = q : q
2 2 1 2 1 2
Thus, we obtain a rule, i.e., if two quantities p and q are in direct proportion, the ratio of any two values of p is
equal to the ratio of the corresponding values of q.

Let’s Attempt

Example 1: Find the value of x and y in the following table, if p and q vary directly.

p 8 4 y
q 20 x 40

Solution: It is given that p and q vary directly, therefore the ratio should be constant.

x
So, 8 4 204 10 and 4 y y 440 16
20 x 8 10 40 10
Example 2: If 20 metres of a uniform copper wire weigh 10 kg, what will be the weight of 6 metres of the
same wire.
Solution: Let the weight of 6 m of wire be x kg, then

Length of wire (in m) (p) 20 6
Weight of wire (in kg) (q) 10 x

It is clear that the lesser the length of the wire, the lesser will be its weight. So, it is a case of direct
proportion. Alternative Approach

The ratio between length of the wire (p) and corresponding meter kg
20 6 20 10
weight (q) is the same. Therefore, =
10 x 6 x
⇒ 20 × x = 6 × 10 [By cross multiplication] Here, x = 6
6 × 10 10 20
⇒ x = = 3 kg
20 610

\ Weight of 6 m wire is 3 kg. or x 20 3kg