Page 30 - Maths Skills - 7

28 Maths

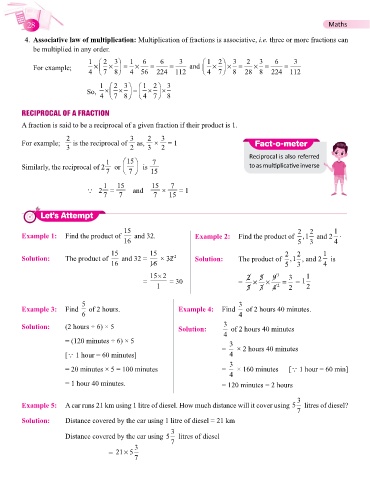
4. Associative law of multiplication: Multiplication of fractions is associative, i.e. three or more fractions can
be multiplied in any order.

1  2 3 1 6 6 3  1 2 3 2 3 6 3
×=
×


For example; 4  7 × 8  = 4 × 56 = 224 = 112 and   4 × 7 ×= 28 8 2224 = 112
8
1  2 3   1 2  3
=
So, ×  ×   ×  ×
4  7 8   4 7  8
RECIPROCAL OF A FRACTION
A fraction is said to be a reciprocal of a given fraction if their product is 1.

2 3 2 3
For example; is the reciprocal of as, × = 1 Fact-o-meter
3 2 3 2
Reciprocal is also referred
1  15  7
Similarly, the reciprocal of 2 or   is to as multiplicative inverse
7  7  15
1 15 15 7
Q 2 = and × = 1
7 7 7 15

Let’s Attempt

15 2 2 1
Example 1: Find the product of and 32. Example 2: Find the product of , 1 and 2 ·
16 5 3 4
15 15 2 2 1
Solution: The product of and 32 = × 32 2 Solution: The product of , 1 , and 2 is
16 16 5 3 4

15 2× 2 5 9 3 3 1
= = 30 = × × = = 1
1 5 3 4 2 2 2

5 3
Example 3: Find of 2 hours. Example 4: Find of 2 hours 40 minutes.
6 4
Solution: (2 hours ÷ 6) × 5 Solution: 3 of 2 hours 40 minutes

= (120 minutes ÷ 6) × 5 4 3
= × 2 hours 40 minutes
[Q 1 hour = 60 minutes] 4
3
= 20 minutes × 5 = 100 minutes = × 160 minutes [Q 1 hour = 60 min]
4
= 1 hour 40 minutes. = 120 minutes = 2 hours

3
Example 5: A car runs 21 km using 1 litre of diesel. How much distance will it cover using 5 litres of diesel?
7
Solution: Distance covered by the car using 1 litre of diesel = 21 km
3
Distance covered by the car using 5 litres of diesel
7
3
= 21 5×
7