Page 11 - Maths Skills - 7

Integers 9

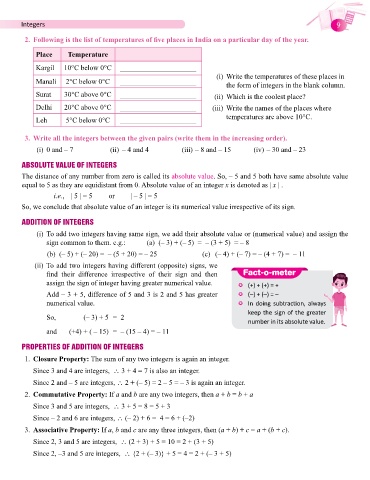
2. Following is the list of temperatures of five places in India on a particular day of the year.

Place Temperature

Kargil 10°C below 0°C _____________________
(i) Write the temperatures of these places in
Manali 2°C below 0°C _____________________ the form of integers in the blank column.
Surat 30°C above 0°C _____________________ (ii) Which is the coolest place?

Delhi 20°C above 0°C _____________________ (iii) Write the names of the places where

Leh 5°C below 0°C _____________________ temperatures are above 10°C.

3. Write all the integers between the given pairs (write them in the increasing order).
(i) 0 and – 7 (ii) – 4 and 4 (iii) – 8 and – 15 (iv) – 30 and – 23

ABSOLUTE VALUE OF INTEGERS
The distance of any number from zero is called its absolute value. So, – 5 and 5 both have same absolute value
equal to 5 as they are equidistant from 0. Absolute value of an integer x is denoted as | x | .
i.e., | 5 | = 5 or | – 5 | = 5

So, we conclude that absolute value of an integer is its numerical value irrespective of its sign.

ADDITION OF INTEGERS
(i) To add two integers having same sign, we add their absolute value or (numerical value) and assign the
sign common to them. e.g.: (a) (– 3) + (– 5) = – (3 + 5) = – 8
(b) (– 5) + (– 20) = – (5 + 20) = – 25 (c) (– 4) + (– 7) = – (4 + 7) = – 11

(ii) To add two integers having different (opposite) signs, we
find their difference irrespective of their sign and then Fact-o-meter
assign the sign of integer having greater numerical value. � (+) + (+) = +

Add – 3 + 5, difference of 5 and 3 is 2 and 5 has greater � (–) + (–) = –
numerical value. � In doing subtraction, always
keep the sign of the greater
So, (– 3) + 5 = 2
number in its absolute value.
and (+4) + ( – 15) = – (15 – 4) = – 11

PROPERTIES OF ADDITION OF INTEGERS

1. Closure Property: The sum of any two integers is again an integer.

Since 3 and 4 are integers, \ 3 + 4 = 7 is also an integer.
Since 2 and – 5 are integers, \ 2 + (– 5) = 2 – 5 = – 3 is again an integer.
2. Commutative Property: If a and b are any two integers, then a + b = b + a

Since 3 and 5 are integers, \ 3 + 5 = 8 = 5 + 3
Since – 2 and 6 are integers, \ (– 2) + 6 = 4 = 6 + (–2)

3. Associative Property: If a, b and c are any three integers, then (a + b) + c = a + (b + c).
Since 2, 3 and 5 are integers, \ (2 + 3) + 5 = 10 = 2 + (3 + 5)
Since 2, –3 and 5 are integers, \ {2 + (– 3)} + 5 = 4 = 2 + (– 3 + 5)