Page 105 - Math Skill - 5

Estimation 103

Rounding off Decimal Quotients
Sometimes, the quotient of a division has a large number of digits after the decimal point. To
make it convenient to calculate or solve problems, we often round off such numbers up to a
certain decimal places. Let us learn through examples.

Let’s Attempt

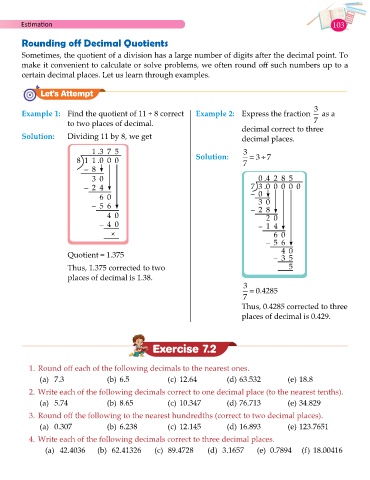
3
Example 1: Find the quotient of 11 ÷ 8 correct Example 2: Express the fraction as a
7
to two places of decimal. decimal correct to three
Solution: Dividing 11 by 8, we get decimal places.

1 .3 7 5 3
37
8 1 1 .0 0 0 Solution: 7 =÷
– 8
3 0 0 .4 2 8 5
– 2 4 7 3 .0 0 0 0 0
6 0 – 0
3 0
– 5 6 – 2 8
4 0 2 0
– 4 0 – 1 4
× 6 0
– 5 6
4 0
Quotient = 1.375 – 3 5
Thus, 1.375 corrected to two 5
places of decimal is 1.38.
3 = 0 4285.
7
Thus, 0.4285 corrected to three
places of decimal is 0.429.

Exercise 7.2

1. Round off each of the following decimals to the nearest ones.
(a) 7.3 (b) 6.5 (c) 12.64 (d) 63.532 (e) 18.8

2. Write each of the following decimals correct to one decimal place (to the nearest tenths).
(a) 5.74 (b) 8.65 (c) 10.347 (d) 76.713 (e) 34.829
3. Round off the following to the nearest hundredths (correct to two decimal places).

(a) 0.307 (b) 6.238 (c) 12.145 (d) 16.893 (e) 123.7651
4. Write each of the following decimals correct to three decimal places.

(a) 42.4036 (b) 62.41326 (c) 89.4728 (d) 3.1657 (e) 0.7894 (f) 18.00416