Page 54 - Mathematics Class - IX

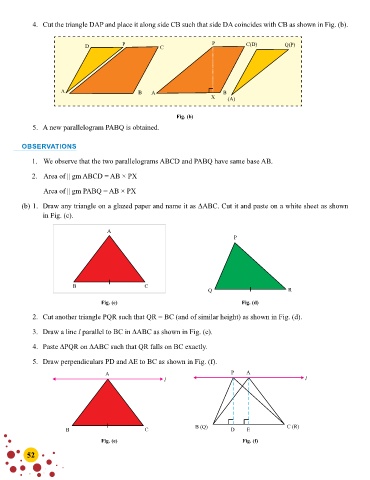
4. Cut the triangle DAP and place it along side CB such that side DA coincides with CB as shown in Fig. (b).

D P C P C(D) Q(P)

A B A B
X (A)

Fig. (b)
5. A new parallelogram PABQ is obtained.

OBSERVATIONS

1. We observe that the two parallelograms ABCD and PABQ have same base AB.

2. Area of || gm ABCD = AB × PX

Area of || gm PABQ = AB × PX

(b) 1. Draw any triangle on a glazed paper and name it as ∆ABC. Cut it and paste on a white sheet as shown
in Fig. (c).

A
P

B C
Q R
Fig. (c) Fig. (d)
2. Cut another triangle PQR such that QR = BC (and of similar height) as shown in Fig. (d).

3. Draw a line l parallel to BC in ∆ABC as shown in Fig. (e).

4. Paste ∆PQR on ∆ABC such that QR falls on BC exactly.

5. Draw perpendiculars PD and AE to BC as shown in Fig. (f).

A P A
l l

B C B (Q) D E C (R)
Fig. (e) Fig. (f)

52