Page 9 - Mathematics Class - XII

Unit I Relations and Functionstions and Functions
Rela

TOPIC - 1: Relations and Functions

ACTIVITY 1.1

OBJECTIVE
To verify that the relation R in the set A of all lines in a plane, defined by R = {(l, m): l ⊥ m} is symmetric but
neither reflexive nor transitive.

MATERIAL REQUIRED

Y A piece of plywood or board Y Glue Y Board pins
Y Some pieces of yarn or thread Y Nails Y White paper

PRE-REQUISITE KNOWLEDGE
1. Knowledge of relations and their types.

2. Knowledge of properties of parallel and perpendicular lines.

PROCEDURE
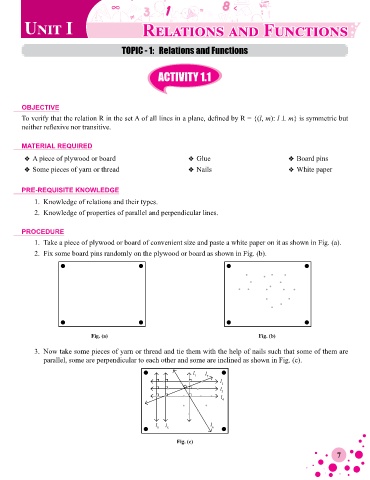
1. Take a piece of plywood or board of convenient size and paste a white paper on it as shown in Fig. (a).
2. Fix some board pins randomly on the plywood or board as shown in Fig. (b).

Fig. (a) Fig. (b)

3. Now take some pieces of yarn or thread and tie them with the help of nails such that some of them are
parallel, some are perpendicular to each other and some are inclined as shown in Fig. (c).

l l
1 7
l 2
l 3
l 4

l l l
8 5 6
Fig. (c)

7