Page 96 - Mathematics Class - XI

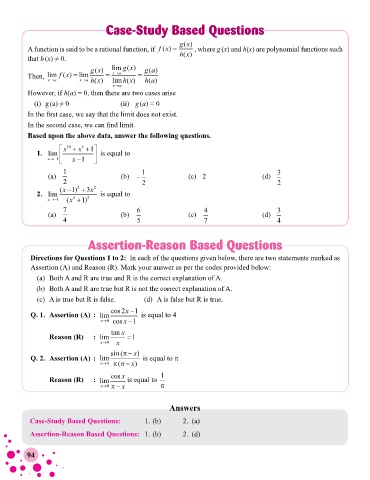
Case-Study Based Questions
Case-Study Based Questions

A function is said to be a rational function, if fx () = gx() , where g (x) and h(x) are polynomial functions such
that h (x) ≠ 0. hx ()
gx
gx lim( ) ga
()
()
Then, lim( )limfx x a
hx
x a x a hx lim( ) ha
()
()
x a
However, if h(a) = 0, then there are two cases arise
(i) g (a) ≠ 0 (ii) g (a) = 0
In the first case, we say that the limit does not exist.
In the second case, we can find limit.
Based upon the above data, answer the following questions.

x x 1
10
5
1. lim is equal to
x 1 x 1
1
(a) 1 (b) − (c) 2 (d) 3
2 2 2
2
2. lim (x 1 ) 3 x 2 is equal to
4
x 1 (x 1 ) 2
(a) 7 (b) 6 (c) 4 (d) 3
4 5 7 4

Assertion-Reason Based Questions
Assertion-Reason Based Questions
Directions for Questions 1 to 2: In each of the questions given below, there are two statements marked as
Assertion (A) and Reason (R). Mark your answer as per the codes provided below:
(a) Both A and R are true and R is the correct explanation of A.
(b) Both A and R are true but R is not the correct explanation of A.

(c) A is true but R is false. (d) A is false but R is true.

cos x 2 1
Q. 1. Assertion (A) : lim is equal to 4
x 0 cos x 1
tan x
Reason (R) : lim 1
x 0 x
sin( ) x
Q. 2. Assertion (A) : lim is equal to p
(
x ) x
Reason (R) : lim cos x is equal to 1
x 0 x p

Answers

Case-Study Based Questions: 1. (b) 2. (a)
Assertion-Reason Based Questions: 1. (b) 2. (d)

94