Page 51 - Mathematics Class - X
P. 51
AB AC AB AC
11. In Fig. (d), = . This gives = . So, BC || QR (by converse of BPT) i.e., ∠B = ∠Q and ∠C = ∠R.
PQ PR BQ CR
Also ∠A = ∠P. That is, the corresponding angles of the two triangles are equal.
Thus, when the corresponding sides of two triangles are proportional, their corresponding angles are equal.
Hence, the two triangles are similar. This is the SSS criterion for similarity of two triangles.
Case 3
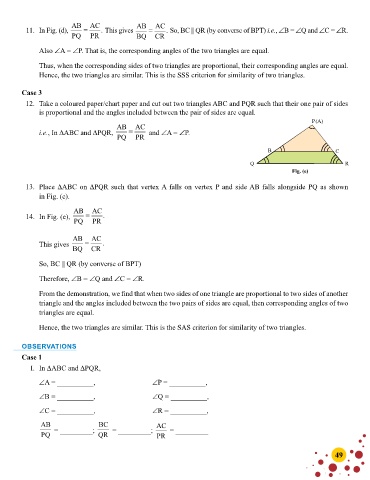
12. Take a coloured paper/chart paper and cut out two triangles ABC and PQR such that their one pair of sides
is proportional and the angles included between the pair of sides are equal.
P (A)
AB AC
i.e., In ∆ABC and ∆PQR, = and ∠A = ∠P.
PQ PR
B C
Q R
Fig. (e)
13. Place ∆ABC on ∆PQR such that vertex A falls on vertex P and side AB falls alongside PQ as shown
in Fig. (e).
AB AC
14. In Fig. (e), = .
PQ PR
AB AC
This gives = .
BQ CR
So, BC || QR (by converse of BPT)
Therefore, ∠B = ∠Q and ∠C = ∠R.
From the demonstration, we find that when two sides of one triangle are proportional to two sides of another
triangle and the angles included between the two pairs of sides are equal, then corresponding angles of two
triangles are equal.
Hence, the two triangles are similar. This is the SAS criterion for similarity of two triangles.
OBSERVATIONS
Case 1
I. In ∆ABC and ∆PQR,
∠A = __________, ∠P = __________,
∠B = __________, ∠Q = __________,
∠C = __________, ∠R = __________,
AB BC AC
PQ = _________; QR = _________; PR = _________
49