Page 61 - Revised Maths Wisdom Class - 6

Number Play 59

Now understand the above concept by using the example given below.
Take a 4-digit number 9264
Now, P = 9642 (biggest number by using digits 9, 2, 6, 4)

Q = 2469 (smallest number by using digits 9, 2, 6, 4)
R = P – Q = 9642 – 2469
R = 7173
Here R ≠ 6174

So, again repeat this concept
Now taking 4-digit number is 7173
Here, P = 7731
Q = 1377

R = P – Q = 7731 – 1377 = 6354
R ≠ 6174
So, again repeat this concept
Now, taking 4-digit number is 6354

Here, P = 6543
Q = 3456
R = P – Q = 6543 – 3456 = 3087
R ≠ 6174

So, again repeat this concept So, again repeat this concept

Now, taking 4-digit number is 3087 Now, taking 4-digit number is 8352
P = 8730 Here, P = 8532
Q = 0378 Q = 2358

R = 8730 – 0378 = 8352 R = P – Q = 8532 – 2358 = 6174
R ≠ 6174 Here, process completed.

The Collatz Conjecture (An Unsolved Mystery)
In 1937, The German Mathematician Lothar Collatz conjectured that the sequence will always reach 1, regardless
the whole number you start with.
According to this conjecture, a sequence is prepared by the rule that one starts with any number. It the number is
even then take half of it and go ahead. But the number is odd then multiply that odd number by 3 and add 1 into
it and again repeat the above process.

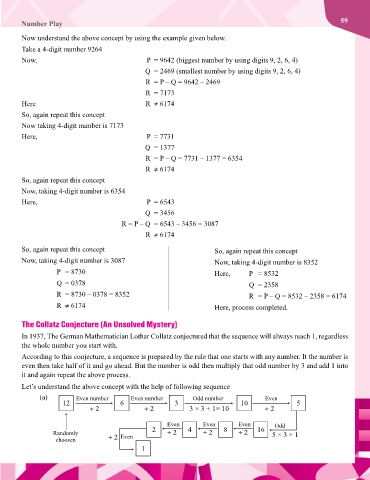
Let’s understand the above concept with the help of following sequence
(a) Even number Even number Odd number Even
12 6 3 10 5
÷ 2 ÷ 2 3 × 3 + 1= 10 ÷ 2

Even Even Even Odd
2 4 8 16
Randomly ÷ 2 ÷ 2 ÷ 2 5 × 3 + 1
choosen ÷ 2 Even
1