Page 12 - Revised Maths Wisdom Class - 6

10 MATHS

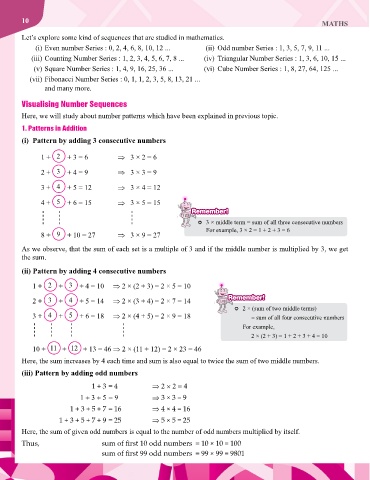
Let’s explore some kind of sequences that are studied in mathematics.
(i) Even number Series : 0, 2, 4, 6, 8, 10, 12 ... (ii) Odd number Series : 1, 3, 5, 7, 9, 11 ...
(iii) Counting Number Series : 1, 2, 3, 4, 5, 6, 7, 8 ... (iv) Triangular Number Series : 1, 3, 6, 10, 15 ...
(v) Square Number Series : 1, 4, 9, 16, 25, 36 ... (vi) Cube Number Series : 1, 8, 27, 64, 125 ...
(vii) Fibonacci Number Series : 0, 1, 1, 2, 3, 5, 8, 13, 21 ...
and many more.

Visualising Number Sequences

Here, we will study about number patterns which have been explained in previous topic.

1. Patterns in Addition
(i) Pattern by adding 3 consecutive numbers

1 + 2 + 3 = 6 ⇒ 3 × 2 = 6

2 + 3 + 4 = 9 ⇒ 3 × 3 = 9

3 + 4 + 5 = 12 ⇒ 3 × 4 = 12

4 + 5 + 6 = 15 ⇒ 3 × 5 = 15
Remember!er!
Rememb
› 3 × middle term = sum of all three consecutive numbers
For example, 3 × 2 = 1 + 2 + 3 = 6
8 + 9 + 10 = 27 ⇒ 3 × 9 = 27
As we observe, that the sum of each set is a multiple of 3 and if the middle number is multiplied by 3, we get
the sum.

(ii) Pattern by adding 4 consecutive numbers

1 + 2 + 3 + 4 = 10 ⇒ 2 × (2 + 3) = 2 × 5 = 10
Rememb
2 + 3 + 4 + 5 = 14 ⇒ 2 × (3 + 4) = 2 × 7 = 14 Remember!er!
› 2 × (sum of two middle terms)
3 + 4 + 5 + 6 = 18 ⇒ 2 × (4 + 5) = 2 × 9 = 18 = sum of all four consecutive numbers
For example,
2 × (2 + 3) = 1 + 2 + 3 + 4 = 10

10 + 11 + 12 + 13 = 46 ⇒ 2 × (11 + 12) = 2 × 23 = 46
Here, the sum increases by 4 each time and sum is also equal to twice the sum of two middle numbers.

(iii) Pattern by adding odd numbers
1 + 3 = 4 ⇒ 2 × 2 = 4

1 + 3 + 5 = 9 ⇒ 3 × 3 = 9
1 + 3 + 5 + 7 = 16 ⇒ 4 × 4 = 16

1 + 3 + 5 + 7 + 9 = 25 ⇒ 5 × 5 = 25
Here, the sum of given odd numbers is equal to the number of odd numbers multiplied by itself.
Thus, sum of first 10 odd numbers = 10 × 10 = 100
sum of first 99 odd numbers = 99 × 99 = 9801