Page 69 - Physics - XII

Terms and Definitions

Spherical Lenses

A piece of refracting medium bounded by at least one spherical surface is called spherical lens. It does not
have a uniform thickness.

Types of Spherical Lenses

There are two types of spherical lenses:

1. Convex or Converging Lenses: These lenses are thickest in the middle and thinner at the edges.
2. Concave or Diverging Lenses: These lense are thinner in the middle than at the edges.
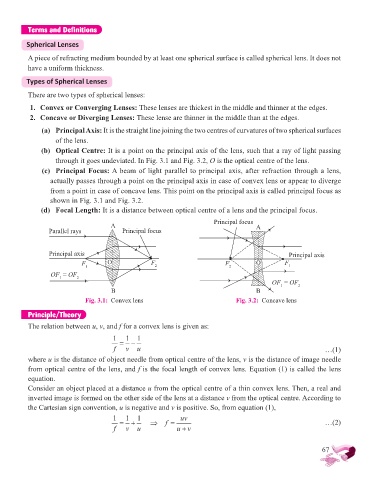
(a) Principal Axis: It is the straight line joining the two centres of curvatures of two spherical surfaces
of the lens.

(b) Optical Centre: It is a point on the principal axis of the lens, such that a ray of light passing
through it goes undeviated. In Fig. 3.1 and Fig. 3.2, O is the optical centre of the lens.
(c) Principal Focus: A beam of light parallel to principal axis, after refraction through a lens,
actually passes through a point on the principal axis in case of convex lens or appear to diverge
from a point in case of concave lens. This point on the principal axis is called principal focus as
shown in Fig. 3.1 and Fig. 3.2.
(d) Focal Length: It is a distance between optical centre of a lens and the principal focus.

Principal focus
A A
Parallel rays Principal focus

Principal axis Principal axis

F O F F O F
1 2 2 1
OF = OF
1 2
OF = OF 2
1
B B
Fig. 3.2: Concave lens
Fig. 3.1: Convex lens Fig. 3.2:
Principle/Theory

The relation between u, v, and f for a convex lens is given as:
1 1 1
f v u …(1)

where u is the distance of object needle from optical centre of the lens, v is the distance of image needle
from optical centre of the lens, and f is the focal length of convex lens. Equation (1) is called the lens
equation.
Consider an object placed at a distance u from the optical centre of a thin convex lens. Then, a real and
inverted image is formed on the other side of the lens at a distance v from the optical centre. According to
the Cartesian sign convention, u is negative and v is positive. So, from equation (1),
1 1 1 f uv …(2)
f v u uv

67