Page 97 - Physics - XI

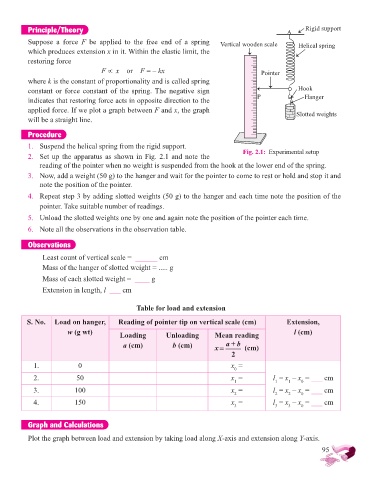
Principle/Theory A Rigid support

Suppose a force F be applied to the free end of a spring Vertical wooden scale Helical spring
which produces extension x in it. Within the elastic limit, the
restoring force 0
F ∝ x or F = – kx 1 Pointer
2
where k is the constant of proportionality and is called spring 3
constant or force constant of the spring. The negative sign 4 Hook
indicates that restoring force acts in opposite direction to the 5 P Hanger
6
applied force. If we plot a graph between F and x, the graph 7 Slotted weights
will be a straight line. 8
9
Procedure 10

1. Suspend the helical spring from the rigid support.
2. Set up the apparatus as shown in Fig. 2.1 and note the Fig. 2.1: Experimental setup
reading of the pointer when no weight is suspended from the hook at the lower end of the spring.
3. Now, add a weight (50 g) to the hanger and wait for the pointer to come to rest or hold and stop it and
note the position of the pointer.
4. Repeat step 3 by adding slotted weights (50 g) to the hanger and each time note the position of the
pointer. Take suitable number of readings.

5. Unload the slotted weights one by one and again note the position of the pointer each time.
6. Note all the observations in the observation table.

Observations
Least count of vertical scale = ______ cm
Mass of the hanger of slotted weight = ..... g

Mass of each slotted weight = ____ g
Extension in length, l ___ cm

Table for load and extension

S. No. Load on hanger, Reading of pointer tip on vertical scale (cm) Extension,
w (g wt) Loading Unloading Mean reading l (cm)

a (cm) b (cm) x = a+ b (cm)
2
1. 0 x =
0
2. 50 x = l = x – x = ___ cm
0
1
1
1
3. 100 x = l = x – x = ___ cm
2
2
0
2
4. 150 x = l = x – x = ___ cm
3
3
3
0
Graph and Calculations
Plot the graph between load and extension by taking load along X-axis and extension along Y-axis.
95