Page 66 - Physics - XI

Q32. In an experiment to study the relation between force of limiting friction and normal reaction,
a body just starts sliding on applying a force of 3 N. What will be the magnitude of the force
of friction acting on the body when the applied forces on it are 0.5 N, 1.0 N, 2.5 N, and 3.5 N,
respectively? (NCERT)
Ans. Given that a body just starts sliding on applying a force of 3 N. So, it is the limiting friction force. It
means if we apply a force less than this force, then the body will not slide. When the applied force
on the body is 0.5 N, the magnitude of the force of friction will also be 0.5 N. When the applied
force on the body is 1.0 N, the magnitude of the force of friction will also be 1.0 N. When the
applied force on the body is 2.5 N, the magnitude of the force of friction will also be 2.5 N. When
the applied force on the body is 3.5 N, the magnitude of the force of friction will be 3 N.

EXPERIMENT - 10

Aim
To fi nd the downward force, along an inclined plane, acting on a roller due to gravitational pull of the earth
and study its relationship with the angle of inclination θ by plotting graph between force and sin θ.

Apparatus and Materials Required
An inclined plane with protractor and pulley, roller, pan, weight box, spring balance, strong thread, half
metre rod, and spirit level

Principle/Theory

Inclined plane
An inclined plane is one of the simplest machine which is used for raising heavy loads by applying less
eff ort. R

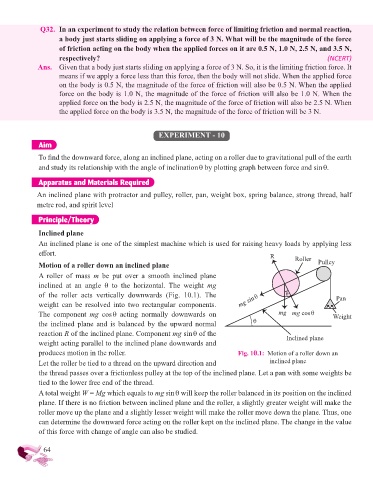
Motion of a roller down an inclined plane Roller Pulley
A roller of mass m be put over a smooth inclined plane
inclined at an angle θ to the horizontal. The weight mg
of the roller acts vertically downwards (Fig. 10.1). The θ Pan
weight can be resolved into two rectangular components. mg sin θ
The component mg cos θ acting normally downwards on mg mg cos θ Weight
the inclined plane and is balanced by the upward normal θ
reaction R of the inclined plane. Component mg sin θ of the
weight acting parallel to the inclined plane downwards and Inclined plane
produces motion in the roller. Fig. 10.1: Motion of a roller down an

Let the roller be tied to a thread on the upward direction and inclined plane
the thread passes over a frictionless pulley at the top of the inclined plane. Let a pan with some weights be
tied to the lower free end of the thread.
A total weight W = Mg which equals to mg sin θ will keep the roller balanced in its position on the inclined
plane. If there is no friction between inclined plane and the roller, a slightly greater weight will make the
roller move up the plane and a slightly lesser weight will make the roller move down the plane. Thus, one
can determine the downward force acting on the roller kept on the inclined plane. The change in the value
of this force with change of angle can also be studied.

64