Page 112 - Physics - XI

Coeffi cient of Viscosity

Due to the relative motion between the diff erent layers of the liquid, a backward dragging force or viscous
force acts tangentially to every layer. The viscous force F is
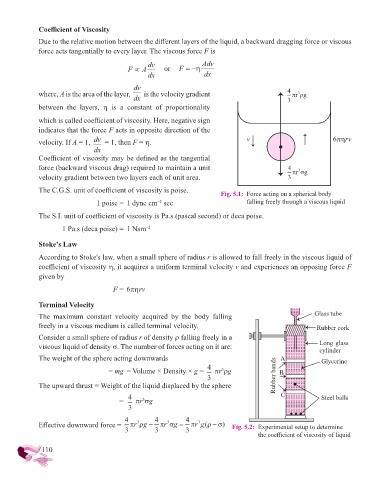
dv Adv
F ∝ A or F
dx dx
dv
where, A is the area of the layer, is the velocity gradient 4 3
dx 3 rg
between the layers, η is a constant of proportionality
which is called coeffi cient of viscosity. Here, negative sign
indicates that the force F acts in opposite direction of the

velocity. If A = 1, dv = 1, then F = η. v 6πηrv
dx
Coeffi cient of viscosity may be defi ned as the tangential
force (backward viscous drag) required to maintain a unit 4 3
velocity gradient between two layers each of unit area. 3 rg

The C.G.S. unit of coeffi cient of viscosity is poise. Fig. 5.1: Force acting on a spherical body

1 poise = 1 dyne cm sec falling freely through a viscous liquid
–2
The S.I. unit of coeffi cient of viscosity is Pa.s (pascal second) or deca poise.

1 Pa.s (deca poise) = 1 Nsm –2

Stoke's Law

According to Stoke's law, when a small sphere of radius r is allowed to fall freely in the viscous liquid of
coeffi cient of viscosity η, it acquires a uniform terminal velocity v and experiences an opposing force F
given by

F = 6πηrv

Terminal Velocity

The maximum constant velocity acquired by the body falling Glass tube
freely in a viscous medium is called terminal velocity. Rubber cork
Consider a small sphere of radius r of density ρ falling freely in a
viscous liquid of density σ. The number of forces acting on it are: Long glass
cylinder
The weight of the sphere acting downwards A Glycerine

= mg = Volume × Density × g = 4 πr ρg B
3
3 Rubber bands
The upward thrust = Weight of the liquid displaced by the sphere

= 4 πr σg C Steel balls
3
3

4
Eff ective downward force = rg 4 rg 4 rg( ) Fig. 5.2: Experimental setup to determine
3
3
3
3 3 3
the coeffi cient of viscosity of liquid
110