Page 204 - Maths Skills - 8

202 Maths

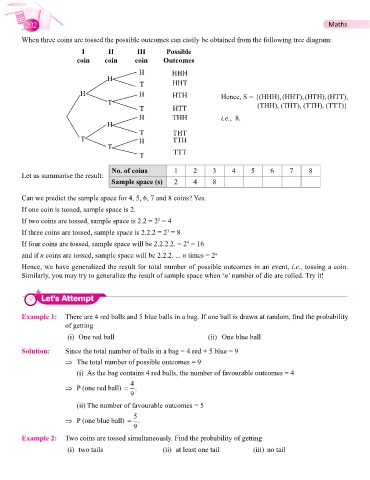
When three coins are tossed the possible outcomes can easily be obtained from the following tree diagram:

I II III Possible
coin coin coin Outcomes

H HHH
H
T HHT
H H HTH Hence, S = {(HHH), (HHT), (HTH), (HTT),
T (THH), (THT), (TTH), (TTT)}
T HTT
H THH i.e., 8.
H
T THT
T H TTH
T
T TTT

No. of coins 1 2 3 4 5 6 7 8
Let us summarise the result:
Sample space (s) 2 4 8

Can we predict the sample space for 4, 5, 6, 7 and 8 coins? Yes.
If one coin is tossed, sample space is 2.
If two coins are tossed, sample space is 2.2 = 2 = 4
2
If three coins are tossed, sample space is 2.2.2 = 2 = 8
3
If four coins are tossed, sample space will be 2.2.2.2. = 2 = 16
4
and if n coins are tossed, sample space will be 2.2.2. ... n times = 2 n
Hence, we have generalized the result for total number of possible outcomes in an event, i.e., tossing a coin.
Similarly, you may try to generalize the result of sample space when ‘n’ number of die are rolled. Try it!

Let’s Attempt

Example 1: There are 4 red balls and 5 blue balls in a bag. If one ball is drawn at random, find the probability
of getting
(i) One red ball (ii) One blue ball

Solution: Since the total number of balls in a bag = 4 red + 5 blue = 9

⇒ The total number of possible outcomes = 9
(i) As the bag contains 4 red balls, the number of favourable outcomes = 4
4
⇒ P (one red ball) = .
9
(ii) The number of favourable outcomes = 5

5
⇒ P (one blue ball) = .
9
Example 2: Two coins are tossed simultaneously. Find the probability of getting

(i) two tails (ii) at least one tail (iii) no tail