Page 158 - Math Skill - 5

156 Maths

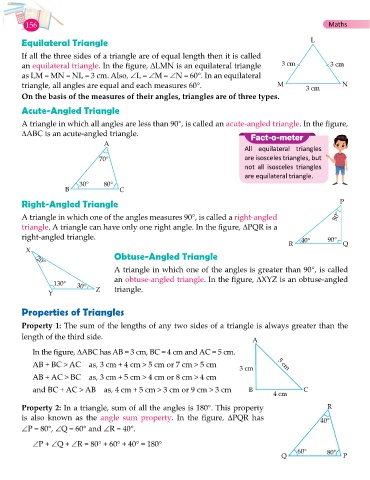
Equilateral Triangle L
If all the three sides of a triangle are of equal length then it is called
an equilateral triangle. In the figure, ∆LMN is an equilateral triangle 3 cm 3 cm
as LM = MN = NL = 3 cm. Also, ∠L = ∠M = ∠N = 60°. In an equilateral
triangle, all angles are equal and each measures 60°. M 3 cm N

On the basis of the measures of their angles, triangles are of three types.

Acute-Angled Triangle
A triangle in which all angles are less than 90°, is called an acute-angled triangle. In the figure,
∆ABC is an acute-angled triangle. Fact-o-meter

A
All equilateral triangles
70° are isosceles triangles, but
not all isosceles triangles
are equilateral triangle.
30° 80°
B C

Right-Angled Triangle P

A triangle in which one of the angles measures 90°, is called a right-angled 50°
triangle. A triangle can have only one right angle. In the figure, ∆PQR is a
right-angled triangle. 90°
R 40° Q
X
Obtuse-Angled Triangle
20°
A triangle in which one of the angles is greater than 90°, is called
an obtuse-angled triangle. In the figure, ∆XYZ is an obtuse-angled
130° 30°
Y Z triangle.

Properties of Triangles

Property 1: The sum of the lengths of any two sides of a triangle is always greater than the
length of the third side. A

In the figure, ∆ABC has AB = 3 cm, BC = 4 cm and AC = 5 cm.

AB + BC > AC as, 3 cm + 4 cm > 5 cm or 7 cm > 5 cm 3 cm 5 cm

AB + AC > BC as, 3 cm + 5 cm > 4 cm or 8 cm > 4 cm
and BC + AC > AB as, 4 cm + 5 cm > 3 cm or 9 cm > 3 cm B C
4 cm

Property 2: In a triangle, sum of all the angles is 180°. This property R
is also known as the angle sum property. In the figure, ∆PQR has 40°
∠P = 80°, ∠Q = 60° and ∠R = 40°.

∠P + ∠Q + ∠R = 80° + 60° + 40° = 180°
60° 80°
Q P