Page 27 - Mathematics Class - XII

DEMONSTRATION
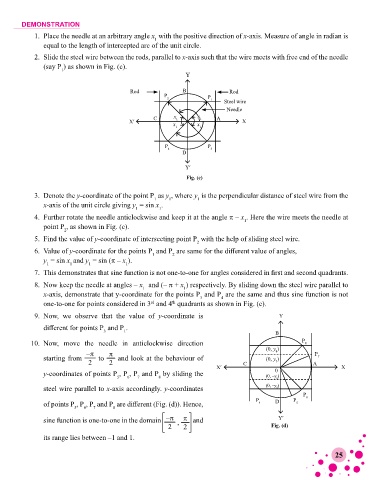
1. Place the needle at an arbitrary angle x with the positive direction of x-axis. Measure of angle in radian is
1
equal to the length of intercepted arc of the unit circle.
2. Slide the steel wire between the rods, parallel to x-axis such that the wire meets with free end of the needle
(say P ) as shown in Fig. (c).
1
Y

Rod B Rod
P 2 P 1 Steel wire

Needle
C x 1 x x 1 1 A
X′ X
x x x
1 1 1
P P
4 3
D

Y′
Fig. (c)

3. Denote the y-coordinate of the point P as y , where y is the perpendicular distance of steel wire from the
1
1
1
x-axis of the unit circle giving y = sin x .
1 1
4. Further rotate the needle anticlockwise and keep it at the angle p – x . Here the wire meets the needle at
1
point P , as shown in Fig. (c).
2
5. Find the value of y-coordinate of intersecting point P with the help of sliding steel wire.
2
6. Value of y-coordinate for the points P and P are same for the different value of angles,
2
1
y = sin x and y = sin (p – x ).
1
1
1
1
7. This demonstrates that sine function is not one-to-one for angles considered in first and second quadrants.
8. Now keep the needle at angles – x and (– p + x ) respectively. By sliding down the steel wire parallel to
1
1
x-axis, demonstrate that y-coordinate for the points P and P are the same and thus sine function is not
3
4
one-to-one for points considered in 3 and 4 quadrants as shown in Fig. (c).
rd
th
9. Now, we observe that the value of y-coordinate is Y
different for points P and P . B
3
1
10. Now, move the needle in anticlockwise direction P 8
–p p (0, y ) P
8
starting from to and look at the behaviour of (0, y ) 7
2 2 C 7 A
X′ X
y-coordinates of points P , P , P and P by sliding the 0
5 6 7 8 (0, –y )
6
steel wire parallel to x-axis accordingly. y-coordinates (0, –y )
5
P 6
of points P , P , P and P are different (Fig. (d)). Hence, P 4 D P 5
7
8
6
5
sine function is one-to-one in the domain –p , p and Y′
2 2 Fig. (d)
its range lies between –1 and 1.
25